ปัญหาปกเวอร์เท็กซ์

สำหรับกราฟที่ไม่มีทิศทาง จุดยอดคือส่วนย่อยของจุดยอด โดยที่ทุกขอบ (u, v) ของกราฟทั้ง u หรือ v อยู่ในชุด

เมื่อใช้ไบนารีทรี เราสามารถแก้ปัญหาจุดยอดได้อย่างง่ายดาย

ปัญหานี้สามารถแบ่งออกเป็นสองปัญหาย่อย เมื่อรากเป็นส่วนหนึ่งของฝาครอบจุดยอด สำหรับกรณีนี้ รูทจะครอบคลุมขอบย่อยทั้งหมด เราสามารถหาขนาดของฝาครอบจุดยอดสำหรับทรีย่อยด้านซ้ายและขวา และเพิ่ม 1 สำหรับรูท

อินพุตและเอาต์พุต

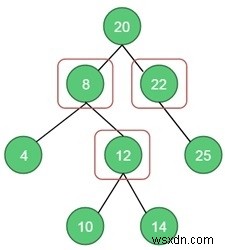
Input:
A binary tree.
Output:
The vertex cover is 3.
 

อัลกอริทึม

vertexCover(root node)

ในปัญหานี้ ไบนารีทรีจะถูกสร้างขึ้น แต่ละโหนดจะเก็บข้อมูลและจำนวนจุดยอดที่ครอบคลุมโดยโหนดนั้น

อินพุต - รากของต้นไม้ไบนารี

ผลลัพธ์ − จำนวนจุดยอดที่รากปกคลุม

Begin
   if root is φ, then
      return 0
   if root has no child, then
      return 0
   if vCover(root) ≠ 0, then
      return vCover(root)
   withRoot := 1 + vertexCover(left(root)) + vertexCover(right(root))
   withoutRoot := 0

   if root has left child, then
      withoutRoot := withoutRoot + vertexCover(left(left(root))) + vertexCover(left(right(root)))
   if root has right child, then
      withoutRoot := withoutRoot + vertexCover(right(left(root))) + vertexCover(right(right(root)))
   return vCover(root)
End

ตัวอย่าง

#include <iostream>
#include <algorithm>
using namespace std;

struct node {
   int data;
   int vCover;
   node *left, *right;
};

node *getNode(int data) {
   node *newNode = new (node);
   newNode->data = data;
   newNode->vCover = 0; //set vertex cover to 0
   newNode->left = NULL;
   newNode->right = NULL;
   return newNode;    //newly created node
}

int vertexCover(node *root) {
   if(root == NULL)    //when tree is empty
      return 0;
   if(root->left == NULL && root->right == NULL)    //when no other edge from root
      return 0;
         
   if(root->vCover != 0)   //when vertex cover of this node is found, leave that node
      return root->vCover;
         
   int sizeWithRoot = 1 + vertexCover(root->left) + vertexCover(root->right);
   int sizeWithOutRoot = 0;
   
   if(root->left != NULL)   //when root is not included and go for left child
      sizeWithOutRoot += 1 + vertexCover(root->left->left) + vertexCover(root->left->right);
   if(root->right != NULL)    //when root is not included and go for right child
      sizeWithOutRoot += 1 + vertexCover(root->right->left) + vertexCover(root->right->right);
   
   root->vCover = (sizeWithRoot < sizeWithOutRoot)?sizeWithRoot:sizeWithOutRoot; //minimum vertex cover
   return root->vCover;
}

int main() {
   //create tree to check vertex cover
   node *root = getNode(20);
   root->left = getNode(8); root->right = getNode(22);
   root->left->left = getNode(4); root->left->right = getNode(12);
   root->left->right->left = getNode(10); root->left->right->right = getNode(14);
   root->right->right = getNode(25);
   cout << "Minimal vertex cover: " << vertexCover(root);
}

ผลลัพธ์

Minimal vertex cover: 3